Class 12 ISC- Java
Class 12th Java aims to empower students by enabling them to build their own applications introducing some effective tools to enable them to enhance their knowledge, broaden horizons, foster creativity, improve the quality of work and increase efficiency.
It also develops logical and analytical thinking so that they can easily solve interactive programs. Students learn fundamental concepts of computing using object oriented approach in one computer language with a clear idea of ethical issues involved in the field of computing
Class 12th java topics includes revision of class 11th, constructors, user-defined methods, objects and classes, library classes , etc.
Karnaugh Maps ( K-Maps )
- It is a graphical representation used for simplifying the boolean expressions.
- For a boolean expression consisting of n-variables, number of blocks required in k-map is 2n cells.
- K-maps are based on Gray codes (Unit distance code).
Types Of K-Maps
K-Map for 2 variables [For SOP Expression] :
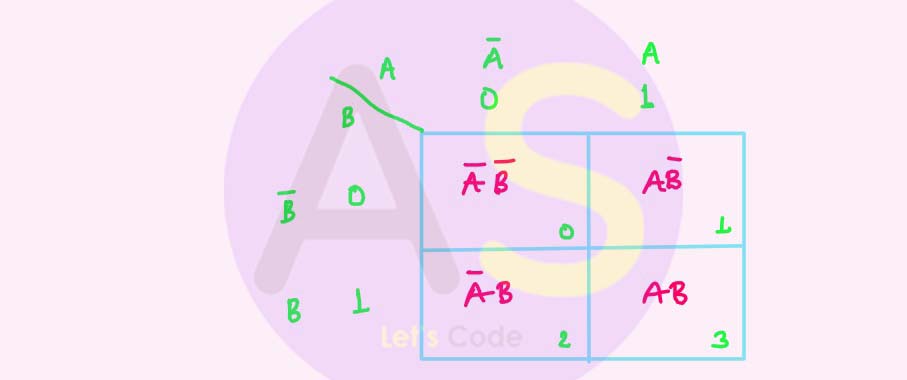

K-Map for 3 variables [For SOP Expression] :
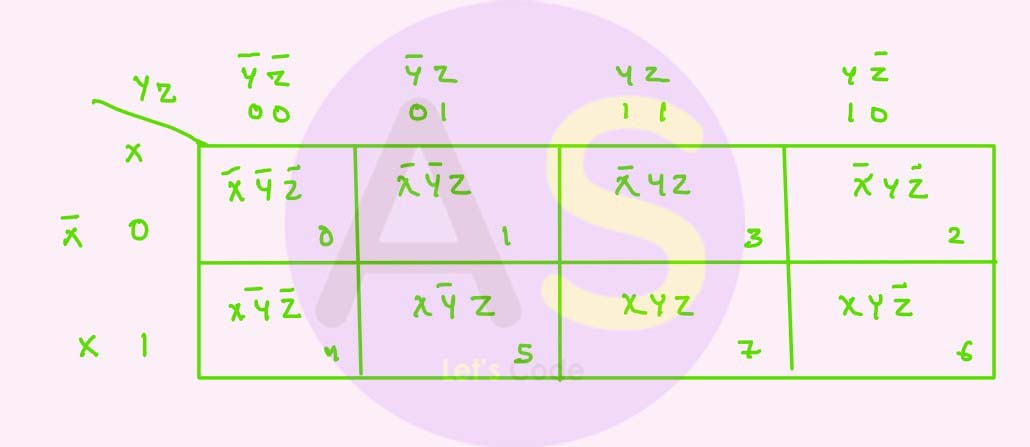

K-Map for 4 variables [For SOP Expression] :
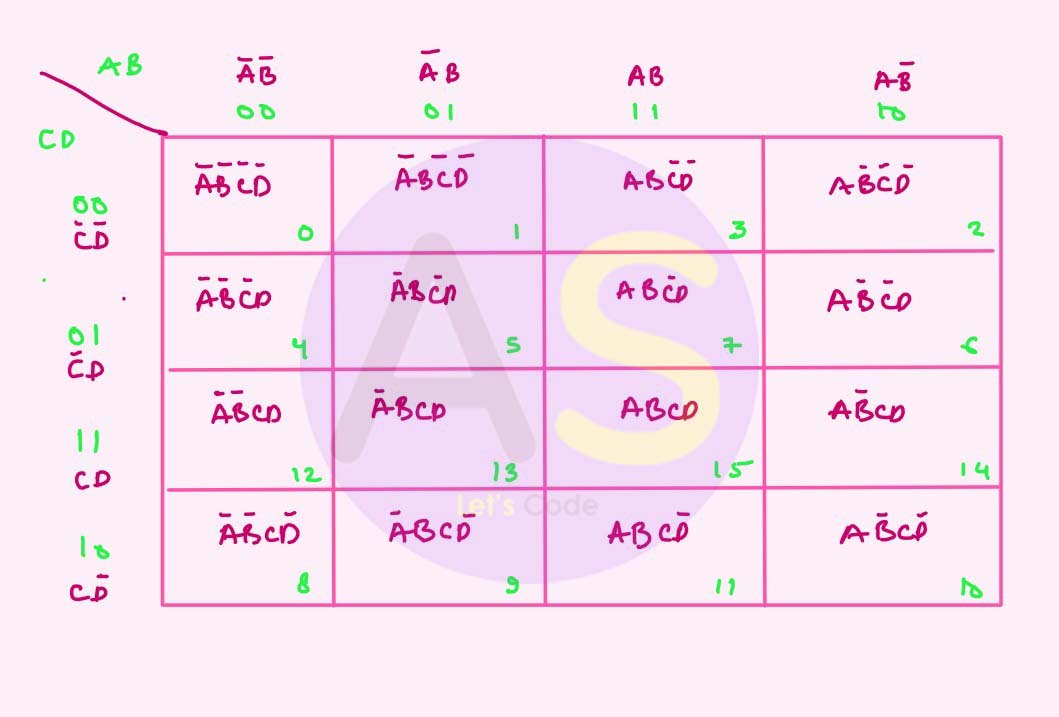

Reducing Boolean expressions through K-Maps
1. Given the Boolean function F(A, B, C, D) = ∑( 0, 2, 3, 4, 5, 8, 10, 11, 12, 13 ).
(i) Reduce the above expression by using 4-variable Karnaugh map, showing the various groups (i.e. octal, quads and pairs).
(ii) Draw the logic gate diagram for the reduced expression Assume that the variables and their complements are available as inputs.
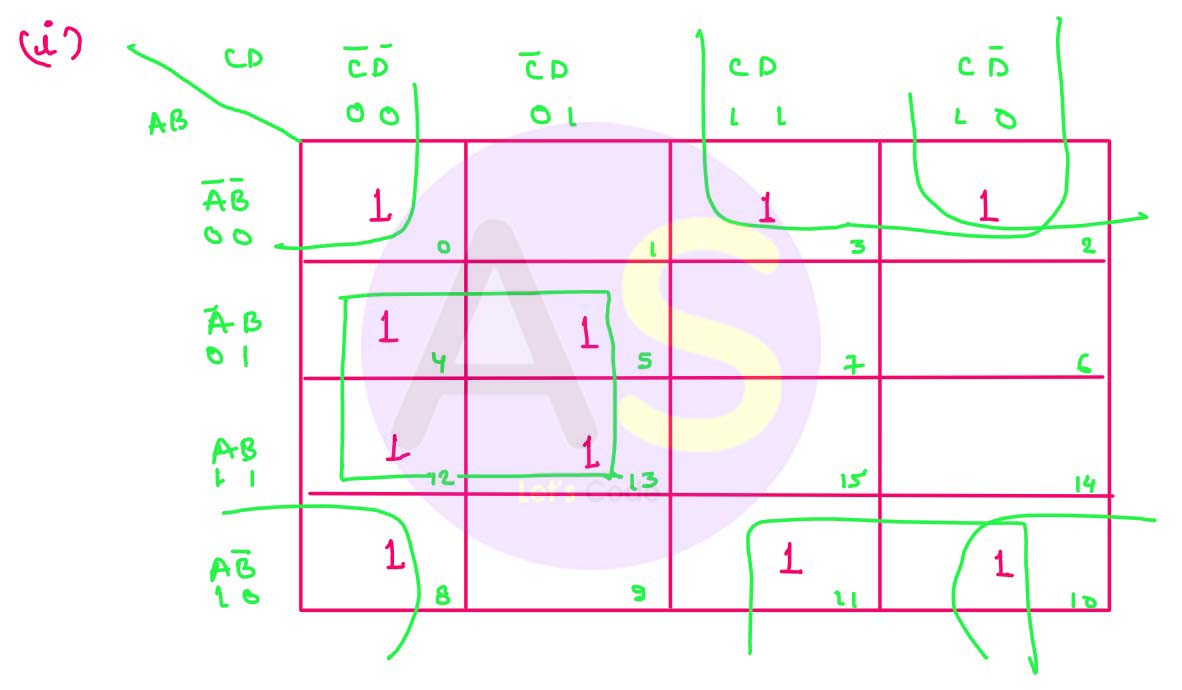
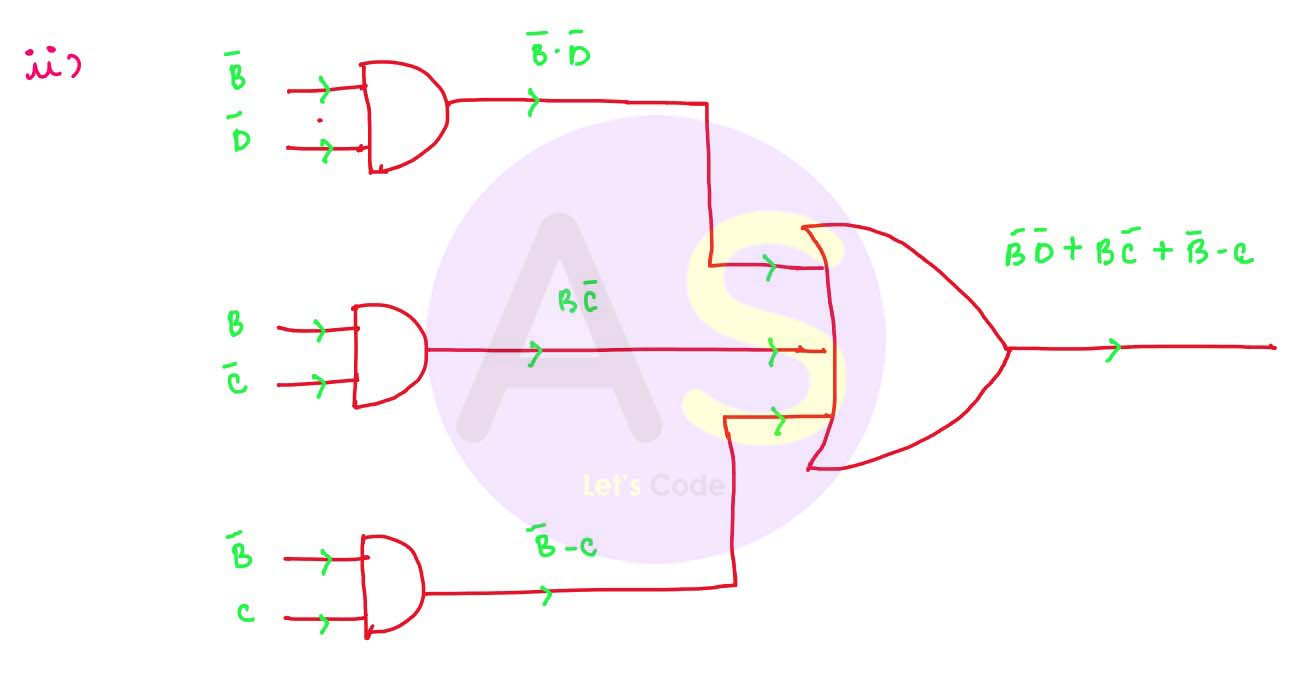
Quad 1 : mo + m2 + m8 + m10 = B'D'
Quad 2 : m4 + m5 + m12 + m13 = BC'
Quad 3 : m3 + m2 + m11 + m10 = B'C
Hence reduced SOP Expressions is : B'D' + BC' + B'C
2. Given the Boolean function F(A,B,C,D) = π( 5, 7, 8, 10, 12, 14, 15 )
(i) Reduce the above expression by using 4-variable Karnaugh map, showing the various groups (i.e. octal, quads and pairs).
(ii) Draw the logic gate diagram for the reduced expression. Assume that the variables and their complements are available as inputs.
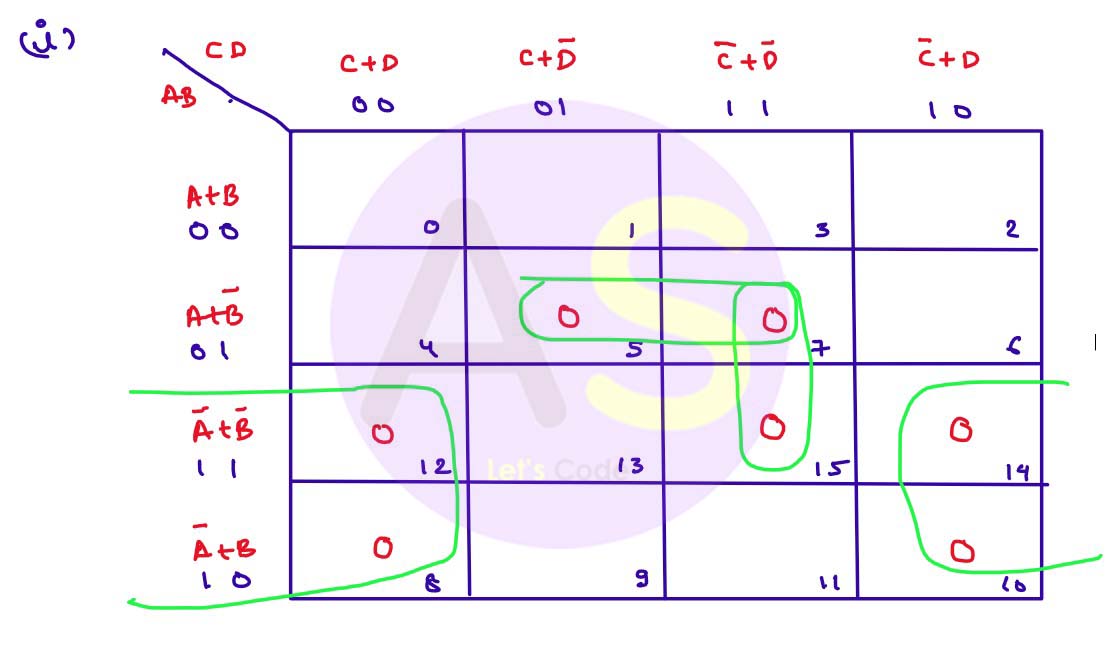
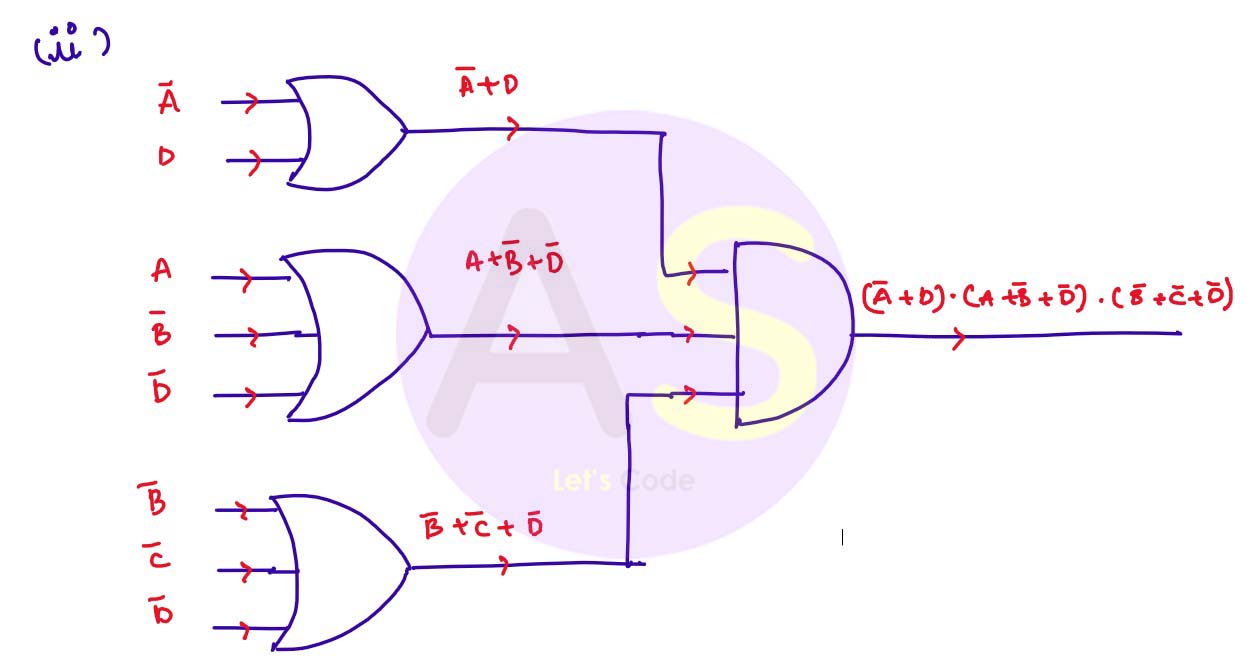
Quad : M8 . M10 . M12 . M14 = A' + D
Pair 1 : M3 . M7 = A + B' + D'
Pair 2 : M7 . M15 = B' + C' + D'
Hence reduced POS Expressions is : (A' + D) . (A + B' + D') . (B' + C' + D')
(i) Reduce the above expression by using 4-variable Karnaugh map, showing the various groups (i.e. octal, quads and pairs).
(ii) Draw the logic gate diagram for the reduced expression Assume that the variables and their complements are available as inputs.

Quad 1 : mo + m2 + m8 + m10 = B'D'
Quad 2 : m4 + m5 + m12 + m13 = BC'
Quad 3 : m3 + m2 + m11 + m10 = B'C
Hence reduced SOP Expressions is : B'D' + BC' + B'C

(i) Reduce the above expression by using 4-variable Karnaugh map, showing the various groups (i.e. octal, quads and pairs).
(ii) Draw the logic gate diagram for the reduced expression. Assume that the variables and their complements are available as inputs.

Quad : M8 . M10 . M12 . M14 = A' + D
Pair 1 : M3 . M7 = A + B' + D'
Pair 2 : M7 . M15 = B' + C' + D'
Hence reduced POS Expressions is : (A' + D) . (A + B' + D') . (B' + C' + D')
